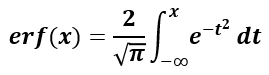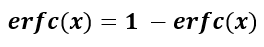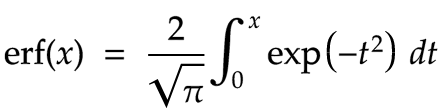Функция ошибок
| Аргумент функции ошибок erf(x) |
| Функция ошибок |
| Дополнительная функция ошибок |
Функция ошибок, она же функция Лапласа, он же интеграл вероятности — все это одна и та же сущность, которая выражается функцией
и используется в статистике и теории вероятностей.
Функция неэлементарная, то есть её нельзя представить в виде элементарных (тригонометрических и алгебраических) функций.
Для расчета в нашем калькуляторе, мы используем связь с неполной гамма функцией
Кроме этого мы сможем здесь же вычислить, дополнительную функцию ошибок, обозначаемую 

В приницпе это все, что можно сказать о ней.
Калькулятор высчитывает результат как в вещественном так и комплексном поле.
Замечание: Функция прекрасно работает на всем поле комплексных чисел при условии если аргумент ( фаза) меньше 180 градусов. Это связано с особенностью вычисления этой функции, неполной гамма функции, интегральной показательной функцией через непрерывные дроби.
Отсюда следует вывод, что при отрицательных вещественных аргументах, функция будет выдавать неверные решения. Но при всех положительных, а также отрицательных комплексных аргументах функция ошибок выдает верный ответ.
Несколько примеров:
About Error Function Calculator
The Error Function Calculator is used to calculate the error function of a given number.
Error Function
In mathematics, the error function is a special function (non-elementary) of sigmoid shape, which occurs in probability, statistics and partial differential equations. It is also called the Gauss error function or probability integral.
The error function is defined as:
Error Function Table
The following is the error function and complementary error function table that shows the values of erf(x) and erfc(x) for x ranging from 0 to 3.5 with an increment of 0.01.
| x | erf(x) | erfc(x) |
|---|---|---|
| 0.0 | 0.0 | 1.0 |
| 0.01 | 0.011283416 | 0.988716584 |
| 0.02 | 0.022564575 | 0.977435425 |
| 0.03 | 0.033841222 | 0.966158778 |
| 0.04 | 0.045111106 | 0.954888894 |
| 0.05 | 0.056371978 | 0.943628022 |
| 0.06 | 0.067621594 | 0.932378406 |
| 0.07 | 0.07885772 | 0.92114228 |
| 0.08 | 0.090078126 | 0.909921874 |
| 0.09 | 0.101280594 | 0.898719406 |
| 0.1 | 0.112462916 | 0.887537084 |
| 0.11 | 0.123622896 | 0.876377104 |
| 0.12 | 0.134758352 | 0.865241648 |
| 0.13 | 0.145867115 | 0.854132885 |
| 0.14 | 0.156947033 | 0.843052967 |
| 0.15 | 0.167995971 | 0.832004029 |
| 0.16 | 0.179011813 | 0.820988187 |
| 0.17 | 0.189992461 | 0.810007539 |
| 0.18 | 0.200935839 | 0.799064161 |
| 0.19 | 0.211839892 | 0.788160108 |
| 0.2 | 0.222702589 | 0.777297411 |
| 0.21 | 0.233521923 | 0.766478077 |
| 0.22 | 0.244295912 | 0.755704088 |
| 0.23 | 0.2550226 | 0.7449774 |
| 0.24 | 0.265700059 | 0.734299941 |
| 0.25 | 0.27632639 | 0.72367361 |
| 0.26 | 0.286899723 | 0.713100277 |
| 0.27 | 0.297418219 | 0.702581781 |
| 0.28 | 0.307880068 | 0.692119932 |
| 0.29 | 0.318283496 | 0.681716504 |
| 0.3 | 0.328626759 | 0.671373241 |
| 0.31 | 0.33890815 | 0.66109185 |
| 0.32 | 0.349125995 | 0.650874005 |
| 0.33 | 0.359278655 | 0.640721345 |
| 0.34 | 0.369364529 | 0.630635471 |
| 0.35 | 0.379382054 | 0.620617946 |
| 0.36 | 0.389329701 | 0.610670299 |
| 0.37 | 0.399205984 | 0.600794016 |
| 0.38 | 0.409009453 | 0.590990547 |
| 0.39 | 0.4187387 | 0.5812613 |
| 0.4 | 0.428392355 | 0.571607645 |
| 0.41 | 0.43796909 | 0.56203091 |
| 0.42 | 0.447467618 | 0.552532382 |
| 0.43 | 0.456886695 | 0.543113305 |
| 0.44 | 0.466225115 | 0.533774885 |
| 0.45 | 0.47548172 | 0.52451828 |
| 0.46 | 0.48465539 | 0.51534461 |
| 0.47 | 0.493745051 | 0.506254949 |
| 0.48 | 0.502749671 | 0.497250329 |
| 0.49 | 0.511668261 | 0.488331739 |
| 0.5 | 0.520499878 | 0.479500122 |
| 0.51 | 0.52924362 | 0.47075638 |
| 0.52 | 0.53789863 | 0.46210137 |
| 0.53 | 0.546464097 | 0.453535903 |
| 0.54 | 0.55493925 | 0.44506075 |
| 0.55 | 0.563323366 | 0.436676634 |
| 0.56 | 0.571615764 | 0.428384236 |
| 0.57 | 0.579815806 | 0.420184194 |
| 0.58 | 0.5879229 | 0.4120771 |
| 0.59 | 0.595936497 | 0.404063503 |
| 0.6 | 0.603856091 | 0.396143909 |
| 0.61 | 0.611681219 | 0.388318781 |
| 0.62 | 0.619411462 | 0.380588538 |
| 0.63 | 0.627046443 | 0.372953557 |
| 0.64 | 0.634585829 | 0.365414171 |
| 0.65 | 0.642029327 | 0.357970673 |
| 0.66 | 0.649376688 | 0.350623312 |
| 0.67 | 0.656627702 | 0.343372298 |
| 0.68 | 0.663782203 | 0.336217797 |
| 0.69 | 0.670840062 | 0.329159938 |
| 0.7 | 0.677801194 | 0.322198806 |
| 0.71 | 0.68466555 | 0.31533445 |
| 0.72 | 0.691433123 | 0.308566877 |
| 0.73 | 0.698103943 | 0.301896057 |
| 0.74 | 0.704678078 | 0.295321922 |
| 0.75 | 0.711155634 | 0.288844366 |
| 0.76 | 0.717536753 | 0.282463247 |
| 0.77 | 0.723821614 | 0.276178386 |
| 0.78 | 0.730010431 | 0.269989569 |
| 0.79 | 0.736103454 | 0.263896546 |
| 0.8 | 0.742100965 | 0.257899035 |
| 0.81 | 0.748003281 | 0.251996719 |
| 0.82 | 0.753810751 | 0.246189249 |
| 0.83 | 0.759523757 | 0.240476243 |
| 0.84 | 0.765142711 | 0.234857289 |
| 0.85 | 0.770668058 | 0.229331942 |
| 0.86 | 0.776100268 | 0.223899732 |
| 0.87 | 0.781439845 | 0.218560155 |
| 0.88 | 0.786687319 | 0.213312681 |
| 0.89 | 0.791843247 | 0.208156753 |
| 0.9 | 0.796908212 | 0.203091788 |
| 0.91 | 0.801882826 | 0.198117174 |
| 0.92 | 0.806767722 | 0.193232278 |
| 0.93 | 0.811563559 | 0.188436441 |
| 0.94 | 0.816271019 | 0.183728981 |
| 0.95 | 0.820890807 | 0.179109193 |
| 0.96 | 0.82542365 | 0.17457635 |
| 0.97 | 0.829870293 | 0.170129707 |
| 0.98 | 0.834231504 | 0.165768496 |
| 0.99 | 0.83850807 | 0.16149193 |
| 1.0 | 0.842700793 | 0.157299207 |
| 1.01 | 0.846810496 | 0.153189504 |
| 1.02 | 0.850838018 | 0.149161982 |
| 1.03 | 0.854784211 | 0.145215789 |
| 1.04 | 0.858649947 | 0.141350053 |
| 1.05 | 0.862436106 | 0.137563894 |
| 1.06 | 0.866143587 | 0.133856413 |
| 1.07 | 0.869773297 | 0.130226703 |
| 1.08 | 0.873326158 | 0.126673842 |
| 1.09 | 0.876803102 | 0.123196898 |
| 1.1 | 0.88020507 | 0.11979493 |
| 1.11 | 0.883533012 | 0.116466988 |
| 1.12 | 0.88678789 | 0.11321211 |
| 1.13 | 0.88997067 | 0.11002933 |
| 1.14 | 0.893082328 | 0.106917672 |
| 1.15 | 0.896123843 | 0.103876157 |
| 1.16 | 0.899096203 | 0.100903797 |
| 1.17 | 0.902000399 | 0.097999601 |
| 1.18 | 0.904837427 | 0.095162573 |
| 1.19 | 0.907608286 | 0.092391714 |
| 1.2 | 0.910313978 | 0.089686022 |
| 1.21 | 0.912955508 | 0.087044492 |
| 1.22 | 0.915533881 | 0.084466119 |
| 1.23 | 0.918050104 | 0.081949896 |
| 1.24 | 0.920505184 | 0.079494816 |
| 1.25 | 0.922900128 | 0.077099872 |
| 1.26 | 0.925235942 | 0.074764058 |
| 1.27 | 0.927513629 | 0.072486371 |
| 1.28 | 0.929734193 | 0.070265807 |
| 1.29 | 0.931898633 | 0.068101367 |
| 1.3 | 0.934007945 | 0.065992055 |
| 1.31 | 0.936063123 | 0.063936877 |
| 1.32 | 0.938065155 | 0.061934845 |
| 1.33 | 0.940015026 | 0.059984974 |
| 1.34 | 0.941913715 | 0.058086285 |
| 1.35 | 0.943762196 | 0.056237804 |
| 1.36 | 0.945561437 | 0.054438563 |
| 1.37 | 0.947312398 | 0.052687602 |
| 1.38 | 0.949016035 | 0.050983965 |
| 1.39 | 0.950673296 | 0.049326704 |
| 1.4 | 0.95228512 | 0.04771488 |
| 1.41 | 0.953852439 | 0.046147561 |
| 1.42 | 0.955376179 | 0.044623821 |
| 1.43 | 0.956857253 | 0.043142747 |
| 1.44 | 0.95829657 | 0.04170343 |
| 1.45 | 0.959695026 | 0.040304974 |
| 1.46 | 0.96105351 | 0.03894649 |
| 1.47 | 0.9623729 | 0.0376271 |
| 1.48 | 0.963654065 | 0.036345935 |
| 1.49 | 0.964897865 | 0.035102135 |
| 1.5 | 0.966105146 | 0.033894854 |
| 1.51 | 0.967276748 | 0.032723252 |
| 1.52 | 0.968413497 | 0.031586503 |
| 1.53 | 0.969516209 | 0.030483791 |
| 1.54 | 0.97058569 | 0.02941431 |
| 1.55 | 0.971622733 | 0.028377267 |
| 1.56 | 0.972628122 | 0.027371878 |
| 1.57 | 0.973602627 | 0.026397373 |
| 1.58 | 0.974547009 | 0.025452991 |
| 1.59 | 0.975462016 | 0.024537984 |
| 1.6 | 0.976348383 | 0.023651617 |
| 1.61 | 0.977206837 | 0.022793163 |
| 1.62 | 0.978038088 | 0.021961912 |
| 1.63 | 0.97884284 | 0.02115716 |
| 1.64 | 0.97962178 | 0.02037822 |
| 1.65 | 0.980375585 | 0.019624415 |
| 1.66 | 0.981104921 | 0.018895079 |
| 1.67 | 0.981810442 | 0.018189558 |
| 1.68 | 0.982492787 | 0.017507213 |
| 1.69 | 0.983152587 | 0.016847413 |
| 1.7 | 0.983790459 | 0.016209541 |
| 1.71 | 0.984407008 | 0.015592992 |
| 1.72 | 0.985002827 | 0.014997173 |
| 1.73 | 0.9855785 | 0.0144215 |
| 1.74 | 0.986134595 | 0.013865405 |
| 1.75 | 0.986671671 | 0.013328329 |
| 1.76 | 0.987190275 | 0.012809725 |
| 1.77 | 0.987690942 | 0.012309058 |
| 1.78 | 0.988174196 | 0.011825804 |
| 1.79 | 0.988640549 | 0.011359451 |
| 1.8 | 0.989090502 | 0.010909498 |
| 1.81 | 0.989524545 | 0.010475455 |
| 1.82 | 0.989943156 | 0.010056844 |
| 1.83 | 0.990346805 | 0.009653195 |
| 1.84 | 0.990735948 | 0.009264052 |
| 1.85 | 0.99111103 | 0.00888897 |
| 1.86 | 0.991472488 | 0.008527512 |
| 1.87 | 0.991820748 | 0.008179252 |
| 1.88 | 0.992156223 | 0.007843777 |
| 1.89 | 0.992479318 | 0.007520682 |
| 1.9 | 0.992790429 | 0.007209571 |
| 1.91 | 0.99308994 | 0.00691006 |
| 1.92 | 0.993378225 | 0.006621775 |
| 1.93 | 0.99365565 | 0.00634435 |
| 1.94 | 0.993922571 | 0.006077429 |
| 1.95 | 0.994179334 | 0.005820666 |
| 1.96 | 0.994426275 | 0.005573725 |
| 1.97 | 0.994663725 | 0.005336275 |
| 1.98 | 0.994892 | 0.005108 |
| 1.99 | 0.995111413 | 0.004888587 |
| 2.0 | 0.995322265 | 0.004677735 |
| 2.01 | 0.995524849 | 0.004475151 |
| 2.02 | 0.995719451 | 0.004280549 |
| 2.03 | 0.995906348 | 0.004093652 |
| 2.04 | 0.99608581 | 0.00391419 |
| 2.05 | 0.996258096 | 0.003741904 |
| 2.06 | 0.996423462 | 0.003576538 |
| 2.07 | 0.996582153 | 0.003417847 |
| 2.08 | 0.996734409 | 0.003265591 |
| 2.09 | 0.996880461 | 0.003119539 |
| 2.1 | 0.997020533 | 0.002979467 |
| 2.11 | 0.997154845 | 0.002845155 |
| 2.12 | 0.997283607 | 0.002716393 |
| 2.13 | 0.997407023 | 0.002592977 |
| 2.14 | 0.997525293 | 0.002474707 |
| 2.15 | 0.997638607 | 0.002361393 |
| 2.16 | 0.997747152 | 0.002252848 |
| 2.17 | 0.997851108 | 0.002148892 |
| 2.18 | 0.997950649 | 0.002049351 |
| 2.19 | 0.998045943 | 0.001954057 |
| 2.2 | 0.998137154 | 0.001862846 |
| 2.21 | 0.998224438 | 0.001775562 |
| 2.22 | 0.998307948 | 0.001692052 |
| 2.23 | 0.998387832 | 0.001612168 |
| 2.24 | 0.998464231 | 0.001535769 |
| 2.25 | 0.998537283 | 0.001462717 |
| 2.26 | 0.998607121 | 0.001392879 |
| 2.27 | 0.998673872 | 0.001326128 |
| 2.28 | 0.998737661 | 0.001262339 |
| 2.29 | 0.998798606 | 0.001201394 |
| 2.3 | 0.998856823 | 0.001143177 |
| 2.31 | 0.998912423 | 0.001087577 |
| 2.32 | 0.998965513 | 0.001034487 |
| 2.33 | 0.999016195 | 0.000983805 |
| 2.34 | 0.99906457 | 0.00093543 |
| 2.35 | 0.999110733 | 0.000889267 |
| 2.36 | 0.999154777 | 0.000845223 |
| 2.37 | 0.99919679 | 0.00080321 |
| 2.38 | 0.999236858 | 0.000763142 |
| 2.39 | 0.999275064 | 0.000724936 |
| 2.4 | 0.999311486 | 0.000688514 |
| 2.41 | 0.999346202 | 0.000653798 |
| 2.42 | 0.999379283 | 0.000620717 |
| 2.43 | 0.999410802 | 0.000589198 |
| 2.44 | 0.999440826 | 0.000559174 |
| 2.45 | 0.99946942 | 0.00053058 |
| 2.46 | 0.999496646 | 0.000503354 |
| 2.47 | 0.999522566 | 0.000477434 |
| 2.48 | 0.999547236 | 0.000452764 |
| 2.49 | 0.999570712 | 0.000429288 |
| 2.5 | 0.999593048 | 0.000406952 |
| 2.51 | 0.999614295 | 0.000385705 |
| 2.52 | 0.999634501 | 0.000365499 |
| 2.53 | 0.999653714 | 0.000346286 |
| 2.54 | 0.999671979 | 0.000328021 |
| 2.55 | 0.99968934 | 0.00031066 |
| 2.56 | 0.999705837 | 0.000294163 |
| 2.57 | 0.999721511 | 0.000278489 |
| 2.58 | 0.9997364 | 0.0002636 |
| 2.59 | 0.999750539 | 0.000249461 |
| 2.6 | 0.999763966 | 0.000236034 |
| 2.61 | 0.999776711 | 0.000223289 |
| 2.62 | 0.999788809 | 0.000211191 |
| 2.63 | 0.999800289 | 0.000199711 |
| 2.64 | 0.999811181 | 0.000188819 |
| 2.65 | 0.999821512 | 0.000178488 |
| 2.66 | 0.999831311 | 0.000168689 |
| 2.67 | 0.999840601 | 0.000159399 |
| 2.68 | 0.999849409 | 0.000150591 |
| 2.69 | 0.999857757 | 0.000142243 |
| 2.7 | 0.999865667 | 0.000134333 |
| 2.71 | 0.999873162 | 0.000126838 |
| 2.72 | 0.999880261 | 0.000119739 |
| 2.73 | 0.999886985 | 0.000113015 |
| 2.74 | 0.999893351 | 0.000106649 |
| 2.75 | 0.999899378 | 0.000100622 |
| 2.76 | 0.999905082 | 9.4918e-05 |
| 2.77 | 0.99991048 | 8.952e-05 |
| 2.78 | 0.999915587 | 8.4413e-05 |
| 2.79 | 0.999920418 | 7.9582e-05 |
| 2.8 | 0.999924987 | 7.5013e-05 |
| 2.81 | 0.999929307 | 7.0693e-05 |
| 2.82 | 0.99993339 | 6.661e-05 |
| 2.83 | 0.99993725 | 6.275e-05 |
| 2.84 | 0.999940898 | 5.9102e-05 |
| 2.85 | 0.999944344 | 5.5656e-05 |
| 2.86 | 0.999947599 | 5.2401e-05 |
| 2.87 | 0.999950673 | 4.9327e-05 |
| 2.88 | 0.999953576 | 4.6424e-05 |
| 2.89 | 0.999956316 | 4.3684e-05 |
| 2.9 | 0.999958902 | 4.1098e-05 |
| 2.91 | 0.999961343 | 3.8657e-05 |
| 2.92 | 0.999963645 | 3.6355e-05 |
| 2.93 | 0.999965817 | 3.4183e-05 |
| 2.94 | 0.999967866 | 3.2134e-05 |
| 2.95 | 0.999969797 | 3.0203e-05 |
| 2.96 | 0.999971618 | 2.8382e-05 |
| 2.97 | 0.999973334 | 2.6666e-05 |
| 2.98 | 0.999974951 | 2.5049e-05 |
| 2.99 | 0.999976474 | 2.3526e-05 |
| 3.0 | 0.99997791 | 2.209e-05 |
| 3.01 | 0.999979261 | 2.0739e-05 |
| 3.02 | 0.999980534 | 1.9466e-05 |
| 3.03 | 0.999981732 | 1.8268e-05 |
| 3.04 | 0.999982859 | 1.7141e-05 |
| 3.05 | 0.99998392 | 1.608e-05 |
| 3.06 | 0.999984918 | 1.5082e-05 |
| 3.07 | 0.999985857 | 1.4143e-05 |
| 3.08 | 0.99998674 | 1.326e-05 |
| 3.09 | 0.999987571 | 1.2429e-05 |
| 3.1 | 0.999988351 | 1.1649e-05 |
| 3.11 | 0.999989085 | 1.0915e-05 |
| 3.12 | 0.999989774 | 1.0226e-05 |
| 3.13 | 0.999990422 | 9.578e-06 |
| 3.14 | 0.99999103 | 8.97e-06 |
| 3.15 | 0.999991602 | 8.398e-06 |
| 3.16 | 0.999992138 | 7.862e-06 |
| 3.17 | 0.999992642 | 7.358e-06 |
| 3.18 | 0.999993115 | 6.885e-06 |
| 3.19 | 0.999993558 | 6.442e-06 |
| 3.2 | 0.999993974 | 6.026e-06 |
| 3.21 | 0.999994365 | 5.635e-06 |
| 3.22 | 0.999994731 | 5.269e-06 |
| 3.23 | 0.999995074 | 4.926e-06 |
| 3.24 | 0.999995396 | 4.604e-06 |
| 3.25 | 0.999995697 | 4.303e-06 |
| 3.26 | 0.99999598 | 4.02e-06 |
| 3.27 | 0.999996245 | 3.755e-06 |
| 3.28 | 0.999996493 | 3.507e-06 |
| 3.29 | 0.999996725 | 3.275e-06 |
| 3.3 | 0.999996942 | 3.058e-06 |
| 3.31 | 0.999997146 | 2.854e-06 |
| 3.32 | 0.999997336 | 2.664e-06 |
| 3.33 | 0.999997515 | 2.485e-06 |
| 3.34 | 0.999997681 | 2.319e-06 |
| 3.35 | 0.999997838 | 2.162e-06 |
| 3.36 | 0.999997983 | 2.017e-06 |
| 3.37 | 0.99999812 | 1.88e-06 |
| 3.38 | 0.999998247 | 1.753e-06 |
| 3.39 | 0.999998367 | 1.633e-06 |
| 3.4 | 0.999998478 | 1.522e-06 |
| 3.41 | 0.999998582 | 1.418e-06 |
| 3.42 | 0.999998679 | 1.321e-06 |
| 3.43 | 0.99999877 | 1.23e-06 |
| 3.44 | 0.999998855 | 1.145e-06 |
| 3.45 | 0.999998934 | 1.066e-06 |
| 3.46 | 0.999999008 | 9.92e-07 |
| 3.47 | 0.999999077 | 9.23e-07 |
| 3.48 | 0.999999141 | 8.59e-07 |
| 3.49 | 0.999999201 | 7.99e-07 |
| 3.5 | 0.999999257 | 7.43e-07 |
See also:
-
Complementary Error Function Calculator
-
Beta Function Calculator
-
Gamma Function Calculator
-
Standard Error Calculator (High Precision)
-
Percentage Error Calculator
-
Proportion Calculator
Frequently Used Miniwebtools:
-
Random Name Picker
-
High-Precision Summation (Sum) Calculator
-
Percent Off Calculator
-
Small Text Generator ⁽ᶜᵒᵖʸ ⁿ ᵖᵃˢᵗᵉ⁾
-
Amortization Calculator
-
Sort Number
-
Ovulation Calendar
-
Average Calculator (High Precision)
-
Cat Years to Human Years Calculator
-
MAC Address Generator
-
ERA Calculator
-
Cm to Feet and Inches Converter
-
Feet and Inches to Cm Converter
-
PVIF Calculator (High Precision)
-
Half Life Calculator
-
Roman Numerals Converter
-
Slugging Percentage Calculator
-
First n Digits of Pi
-
Mean Absolute Deviation Calculator
-
KPa to Psi Converter
-
Cube Root Calculator
-
Psi to KPa Converter
-
Area of a Trapezoid Calculator
-
Surface Area of a Rectangular Prism Calculator (High Precision)
-
Ellipse Circumference Calculator
-
Day of Year Calendar
-
Feet to Meters Converter
-
Hex Calculator
-
Surface Area of a Cone Calculator (High Precision)
-
Random Quote Generator
-
Fixed Asset Turnover Calculator
-
Equivalent Fractions Calculator
-
Error Function Calculator
-
Antilog Calculator
-
Coefficient of Variation Calculator
-
PPM to Percent Converter
-
Sum of Squares Calculator
-
Golden Rectangle Calculator
-
Salary Conversion Calculator
-
Area of a Parallelogram Calculator
-
Leap Years List
-
Midrange Calculator
-
Prime Factor Calculator
-
Batting Average Calculator
-
Doubling Time Calculator
-
10 Minutes
-
Bitwise Calculator
-
Word to Phone Number Converter
-
Cube Numbers List
-
Acid Test Ratio Calculator
-
Total Asset Turnover Calculator
-
Area of A Sector Calculator
-
Line Counter
-
Volume of a Rectangular Prism Calculator (High Precision)
-
Surface Area of Sphere Calculator (High Precision)
-
Sum of Positive Integers Calculator
-
Relative Standard Deviation Calculator (High Precision)
-
On Base Percentage Calculator
-
Log Base 2 Calculator
-
Binary to Gray Code Converter
-
Log Base 10 Calculator
-
Square Numbers List
-
Phone Number Extractor
-
Gray Code to Binary Converter
-
Present Value of Annuity Calculator
-
PVIFA Calculator (High Precision)
-
Binary Calculator
-
Percent to PPM Converter
-
Remove Spaces
| Error function | |
|---|---|
Plot of the error function |
|
| General information | |
| General definition |  |
| Fields of application | Probability, thermodynamics |
| Domain, Codomain and Image | |
| Domain |  |
| Image |  |
| Basic features | |
| Parity | Odd |
| Specific features | |
| Root | 0 |
| Derivative |  |
| Antiderivative |  |
| Series definition | |
| Taylor series |  |
In mathematics, the error function (also called the Gauss error function), often denoted by erf, is a complex function of a complex variable defined as:[1]
Some authors define 

This nonelementary integral is a sigmoid function that occurs often in probability, statistics, and partial differential equations. In many of these applications, the function argument is a real number. If the function argument is real, then the function value is also real.
In statistics, for non-negative values of x, the error function has the following interpretation: for a random variable Y that is normally distributed with mean 0 and standard deviation 1/√2, erf x is the probability that Y falls in the range [−x, x].
Two closely related functions are the complementary error function (erfc) defined as
and the imaginary error function (erfi) defined as
where i is the imaginary unit.
Name[edit]
The name «error function» and its abbreviation erf were proposed by J. W. L. Glaisher in 1871 on account of its connection with «the theory of Probability, and notably the theory of Errors.»[3] The error function complement was also discussed by Glaisher in a separate publication in the same year.[4]
For the «law of facility» of errors whose density is given by
(the normal distribution), Glaisher calculates the probability of an error lying between p and q as:
Plot of the error function Erf(z) in the complex plane from -2-2i to 2+2i with colors created with Mathematica 13.1 function ComplexPlot3D
Applications[edit]
When the results of a series of measurements are described by a normal distribution with standard deviation σ and expected value 0, then erf (a/σ √2) is the probability that the error of a single measurement lies between −a and +a, for positive a. This is useful, for example, in determining the bit error rate of a digital communication system.
The error and complementary error functions occur, for example, in solutions of the heat equation when boundary conditions are given by the Heaviside step function.
The error function and its approximations can be used to estimate results that hold with high probability or with low probability. Given a random variable X ~ Norm[μ,σ] (a normal distribution with mean μ and standard deviation σ) and a constant L < μ:
where A and B are certain numeric constants. If L is sufficiently far from the mean, specifically μ − L ≥ σ√ln k, then:
so the probability goes to 0 as k → ∞.
The probability for X being in the interval [La, Lb] can be derived as
Properties[edit]
Integrand exp(−z2)
erf z
The property erf (−z) = −erf z means that the error function is an odd function. This directly results from the fact that the integrand e−t2 is an even function (the antiderivative of an even function which is zero at the origin is an odd function and vice versa).
Since the error function is an entire function which takes real numbers to real numbers, for any complex number z:
where z is the complex conjugate of z.
The integrand f = exp(−z2) and f = erf z are shown in the complex z-plane in the figures at right with domain coloring.
The error function at +∞ is exactly 1 (see Gaussian integral). At the real axis, erf z approaches unity at z → +∞ and −1 at z → −∞. At the imaginary axis, it tends to ±i∞.
Taylor series[edit]
The error function is an entire function; it has no singularities (except that at infinity) and its Taylor expansion always converges, but is famously known «[…] for its bad convergence if x > 1.»[5]
The defining integral cannot be evaluated in closed form in terms of elementary functions (see Liouville’s theorem), but by expanding the integrand e−z2 into its Maclaurin series and integrating term by term, one obtains the error function’s Maclaurin series as:
which holds for every complex number z. The denominator terms are sequence A007680 in the OEIS.
For iterative calculation of the above series, the following alternative formulation may be useful:
because −(2k − 1)z2/k(2k + 1) expresses the multiplier to turn the kth term into the (k + 1)th term (considering z as the first term).
The imaginary error function has a very similar Maclaurin series, which is:
which holds for every complex number z.
Derivative and integral[edit]
The derivative of the error function follows immediately from its definition:
From this, the derivative of the imaginary error function is also immediate:
An antiderivative of the error function, obtainable by integration by parts, is
An antiderivative of the imaginary error function, also obtainable by integration by parts, is
Higher order derivatives are given by
where H are the physicists’ Hermite polynomials.[6]
Bürmann series[edit]
An expansion,[7] which converges more rapidly for all real values of x than a Taylor expansion, is obtained by using Hans Heinrich Bürmann’s theorem:[8]
where sgn is the sign function. By keeping only the first two coefficients and choosing c1 = 31/200 and c2 = −341/8000, the resulting approximation shows its largest relative error at x = ±1.3796, where it is less than 0.0036127:
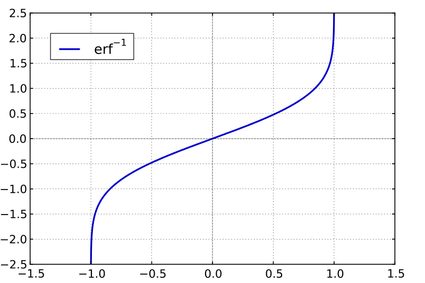
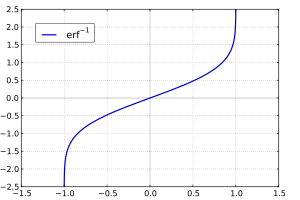
Inverse functions[edit]
Given a complex number z, there is not a unique complex number w satisfying erf w = z, so a true inverse function would be multivalued. However, for −1 < x < 1, there is a unique real number denoted erf−1 x satisfying
The inverse error function is usually defined with domain (−1,1), and it is restricted to this domain in many computer algebra systems. However, it can be extended to the disk |z| < 1 of the complex plane, using the Maclaurin series[9]
where c0 = 1 and
So we have the series expansion (common factors have been canceled from numerators and denominators):
(After cancellation the numerator/denominator fractions are entries OEIS: A092676/OEIS: A092677 in the OEIS; without cancellation the numerator terms are given in entry OEIS: A002067.) The error function’s value at ±∞ is equal to ±1.
For |z| < 1, we have erf(erf−1 z) = z.
The inverse complementary error function is defined as
For real x, there is a unique real number erfi−1 x satisfying erfi(erfi−1 x) = x. The inverse imaginary error function is defined as erfi−1 x.[10]
For any real x, Newton’s method can be used to compute erfi−1 x, and for −1 ≤ x ≤ 1, the following Maclaurin series converges:
where ck is defined as above.
Asymptotic expansion[edit]
A useful asymptotic expansion of the complementary error function (and therefore also of the error function) for large real x is
where (2n − 1)!! is the double factorial of (2n − 1), which is the product of all odd numbers up to (2n − 1). This series diverges for every finite x, and its meaning as asymptotic expansion is that for any integer N ≥ 1 one has
where the remainder is
which follows easily by induction, writing
and integrating by parts.
The asymptotic behavior of the remainder term, in Landau notation, is
as x → ∞. This can be found by
For large enough values of x, only the first few terms of this asymptotic expansion are needed to obtain a good approximation of erfc x (while for not too large values of x, the above Taylor expansion at 0 provides a very fast convergence).
Continued fraction expansion[edit]
A continued fraction expansion of the complementary error function is:[11]
Integral of error function with Gaussian density function[edit]
which appears related to Ng and Geller, formula 13 in section 4.3[12] with a change of variables.
Factorial series[edit]
The inverse factorial series:
converges for Re(z2) > 0. Here
zn denotes the rising factorial, and s(n,k) denotes a signed Stirling number of the first kind.[13][14]
There also exists a representation by an infinite sum containing the double factorial:
Numerical approximations[edit]
Approximation with elementary functions[edit]
- Abramowitz and Stegun give several approximations of varying accuracy (equations 7.1.25–28). This allows one to choose the fastest approximation suitable for a given application. In order of increasing accuracy, they are:
(maximum error: 5×10−4)
where a1 = 0.278393, a2 = 0.230389, a3 = 0.000972, a4 = 0.078108
(maximum error: 2.5×10−5)
where p = 0.47047, a1 = 0.3480242, a2 = −0.0958798, a3 = 0.7478556
(maximum error: 3×10−7)
where a1 = 0.0705230784, a2 = 0.0422820123, a3 = 0.0092705272, a4 = 0.0001520143, a5 = 0.0002765672, a6 = 0.0000430638
(maximum error: 1.5×10−7)
where p = 0.3275911, a1 = 0.254829592, a2 = −0.284496736, a3 = 1.421413741, a4 = −1.453152027, a5 = 1.061405429
All of these approximations are valid for x ≥ 0. To use these approximations for negative x, use the fact that erf x is an odd function, so erf x = −erf(−x).
- Exponential bounds and a pure exponential approximation for the complementary error function are given by[15]
- The above have been generalized to sums of N exponentials[16] with increasing accuracy in terms of N so that erfc x can be accurately approximated or bounded by 2Q̃(√2x), where
In particular, there is a systematic methodology to solve the numerical coefficients {(an,bn)}N
n = 1 that yield a minimax approximation or bound for the closely related Q-function: Q(x) ≈ Q̃(x), Q(x) ≤ Q̃(x), or Q(x) ≥ Q̃(x) for x ≥ 0. The coefficients {(an,bn)}N
n = 1 for many variations of the exponential approximations and bounds up to N = 25 have been released to open access as a comprehensive dataset.[17] - A tight approximation of the complementary error function for x ∈ [0,∞) is given by Karagiannidis & Lioumpas (2007)[18] who showed for the appropriate choice of parameters {A,B} that
They determined {A,B} = {1.98,1.135}, which gave a good approximation for all x ≥ 0. Alternative coefficients are also available for tailoring accuracy for a specific application or transforming the expression into a tight bound.[19]
- A single-term lower bound is[20]
where the parameter β can be picked to minimize error on the desired interval of approximation.
-
- Another approximation is given by Sergei Winitzki using his «global Padé approximations»:[21][22]: 2–3
where
This is designed to be very accurate in a neighborhood of 0 and a neighborhood of infinity, and the relative error is less than 0.00035 for all real x. Using the alternate value a ≈ 0.147 reduces the maximum relative error to about 0.00013.[23]
This approximation can be inverted to obtain an approximation for the inverse error function:
- An approximation with a maximal error of 1.2×10−7 for any real argument is:[24]
with
and
- An approximation of
with a maximum relative error less than
in absolute value is:[25]
for
,
and for
Table of values[edit]
| x | erf x | 1 − erf x |
|---|---|---|
| 0 | 0 | 1 |
| 0.02 | 0.022564575 | 0.977435425 |
| 0.04 | 0.045111106 | 0.954888894 |
| 0.06 | 0.067621594 | 0.932378406 |
| 0.08 | 0.090078126 | 0.909921874 |
| 0.1 | 0.112462916 | 0.887537084 |
| 0.2 | 0.222702589 | 0.777297411 |
| 0.3 | 0.328626759 | 0.671373241 |
| 0.4 | 0.428392355 | 0.571607645 |
| 0.5 | 0.520499878 | 0.479500122 |
| 0.6 | 0.603856091 | 0.396143909 |
| 0.7 | 0.677801194 | 0.322198806 |
| 0.8 | 0.742100965 | 0.257899035 |
| 0.9 | 0.796908212 | 0.203091788 |
| 1 | 0.842700793 | 0.157299207 |
| 1.1 | 0.880205070 | 0.119794930 |
| 1.2 | 0.910313978 | 0.089686022 |
| 1.3 | 0.934007945 | 0.065992055 |
| 1.4 | 0.952285120 | 0.047714880 |
| 1.5 | 0.966105146 | 0.033894854 |
| 1.6 | 0.976348383 | 0.023651617 |
| 1.7 | 0.983790459 | 0.016209541 |
| 1.8 | 0.989090502 | 0.010909498 |
| 1.9 | 0.992790429 | 0.007209571 |
| 2 | 0.995322265 | 0.004677735 |
| 2.1 | 0.997020533 | 0.002979467 |
| 2.2 | 0.998137154 | 0.001862846 |
| 2.3 | 0.998856823 | 0.001143177 |
| 2.4 | 0.999311486 | 0.000688514 |
| 2.5 | 0.999593048 | 0.000406952 |
| 3 | 0.999977910 | 0.000022090 |
| 3.5 | 0.999999257 | 0.000000743 |
[edit]
Complementary error function[edit]
The complementary error function, denoted erfc, is defined as
Plot of the complementary error function Erfc(z) in the complex plane from -2-2i to 2+2i with colors created with Mathematica 13.1 function ComplexPlot3D
which also defines erfcx, the scaled complementary error function[26] (which can be used instead of erfc to avoid arithmetic underflow[26][27]). Another form of erfc x for x ≥ 0 is known as Craig’s formula, after its discoverer:[28]
This expression is valid only for positive values of x, but it can be used in conjunction with erfc x = 2 − erfc(−x) to obtain erfc(x) for negative values. This form is advantageous in that the range of integration is fixed and finite. An extension of this expression for the erfc of the sum of two non-negative variables is as follows:[29]
Imaginary error function[edit]
The imaginary error function, denoted erfi, is defined as
where D(x) is the Dawson function (which can be used instead of erfi to avoid arithmetic overflow[26]).
Despite the name «imaginary error function», erfi x is real when x is real.
When the error function is evaluated for arbitrary complex arguments z, the resulting complex error function is usually discussed in scaled form as the Faddeeva function:
Cumulative distribution function[edit]
The error function is essentially identical to the standard normal cumulative distribution function, denoted Φ, also named norm(x) by some software languages[citation needed], as they differ only by scaling and translation. Indeed,
the normal cumulative distribution function plotted in the complex plane
or rearranged for erf and erfc:
Consequently, the error function is also closely related to the Q-function, which is the tail probability of the standard normal distribution. The Q-function can be expressed in terms of the error function as
The inverse of Φ is known as the normal quantile function, or probit function and may be expressed in terms of the inverse error function as
The standard normal cdf is used more often in probability and statistics, and the error function is used more often in other branches of mathematics.
The error function is a special case of the Mittag-Leffler function, and can also be expressed as a confluent hypergeometric function (Kummer’s function):
It has a simple expression in terms of the Fresnel integral.[further explanation needed]
In terms of the regularized gamma function P and the incomplete gamma function,
sgn x is the sign function.
Generalized error functions[edit]
grey curve: E1(x) = 1 − e−x/√π
red curve: E2(x) = erf(x)
green curve: E3(x)
blue curve: E4(x)
gold curve: E5(x).
Some authors discuss the more general functions:[citation needed]
Notable cases are:
- E0(x) is a straight line through the origin: E0(x) = x/e√π
- E2(x) is the error function, erf x.
After division by n!, all the En for odd n look similar (but not identical) to each other. Similarly, the En for even n look similar (but not identical) to each other after a simple division by n!. All generalised error functions for n > 0 look similar on the positive x side of the graph.
These generalised functions can equivalently be expressed for x > 0 using the gamma function and incomplete gamma function:
Therefore, we can define the error function in terms of the incomplete gamma function:
Iterated integrals of the complementary error function[edit]
The iterated integrals of the complementary error function are defined by[30]
The general recurrence formula is
They have the power series
from which follow the symmetry properties
and
Implementations[edit]
As real function of a real argument[edit]
- In POSIX-compliant operating systems, the header
math.hshall declare and the mathematical librarylibmshall provide the functionserfanderfc(double precision) as well as their single precision and extended precision counterpartserff,erflanderfcf,erfcl.[31]
- The GNU Scientific Library provides
erf,erfc,log(erf), and scaled error functions.[32]
As complex function of a complex argument[edit]
libcerf, numeric C library for complex error functions, provides the complex functionscerf,cerfc,cerfcxand the real functionserfi,erfcxwith approximately 13–14 digits precision, based on the Faddeeva function as implemented in the MIT Faddeeva Package
See also[edit]
[edit]
- Gaussian integral, over the whole real line
- Gaussian function, derivative
- Dawson function, renormalized imaginary error function
- Goodwin–Staton integral
In probability[edit]
- Normal distribution
- Normal cumulative distribution function, a scaled and shifted form of error function
- Probit, the inverse or quantile function of the normal CDF
- Q-function, the tail probability of the normal distribution
- Standard score
References[edit]
- ^ Andrews, Larry C. (1998). Special functions of mathematics for engineers. SPIE Press. p. 110. ISBN 9780819426161.
- ^ Whittaker, E. T.; Watson, G. N. (1927). A Course of Modern Analysis. Cambridge University Press. p. 341. ISBN 978-0-521-58807-2.
- ^ Glaisher, James Whitbread Lee (July 1871). «On a class of definite integrals». London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science. 4. 42 (277): 294–302. doi:10.1080/14786447108640568. Retrieved 6 December 2017.
- ^ Glaisher, James Whitbread Lee (September 1871). «On a class of definite integrals. Part II». London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science. 4. 42 (279): 421–436. doi:10.1080/14786447108640600. Retrieved 6 December 2017.
- ^ «A007680 – OEIS». oeis.org. Retrieved 2 April 2020.
- ^ Weisstein, Eric W. «Erf». MathWorld.
- ^ Schöpf, H. M.; Supancic, P. H. (2014). «On Bürmann’s Theorem and Its Application to Problems of Linear and Nonlinear Heat Transfer and Diffusion». The Mathematica Journal. 16. doi:10.3888/tmj.16-11.
- ^ Weisstein, Eric W. «Bürmann’s Theorem». MathWorld.
- ^ Dominici, Diego (2006). «Asymptotic analysis of the derivatives of the inverse error function». arXiv:math/0607230.
- ^ Bergsma, Wicher (2006). «On a new correlation coefficient, its orthogonal decomposition and associated tests of independence». arXiv:math/0604627.
- ^ Cuyt, Annie A. M.; Petersen, Vigdis B.; Verdonk, Brigitte; Waadeland, Haakon; Jones, William B. (2008). Handbook of Continued Fractions for Special Functions. Springer-Verlag. ISBN 978-1-4020-6948-2.
- ^ Ng, Edward W.; Geller, Murray (January 1969). «A table of integrals of the Error functions». Journal of Research of the National Bureau of Standards Section B. 73B (1): 1. doi:10.6028/jres.073B.001.
- ^ Schlömilch, Oskar Xavier (1859). «Ueber facultätenreihen». Zeitschrift für Mathematik und Physik (in German). 4: 390–415.
- ^ Nielson, Niels (1906). Handbuch der Theorie der Gammafunktion (in German). Leipzig: B. G. Teubner. p. 283 Eq. 3. Retrieved 4 December 2017.
- ^ Chiani, M.; Dardari, D.; Simon, M.K. (2003). «New Exponential Bounds and Approximations for the Computation of Error Probability in Fading Channels» (PDF). IEEE Transactions on Wireless Communications. 2 (4): 840–845. CiteSeerX 10.1.1.190.6761. doi:10.1109/TWC.2003.814350.
- ^ Tanash, I.M.; Riihonen, T. (2020). «Global minimax approximations and bounds for the Gaussian Q-function by sums of exponentials». IEEE Transactions on Communications. 68 (10): 6514–6524. arXiv:2007.06939. doi:10.1109/TCOMM.2020.3006902. S2CID 220514754.
- ^ Tanash, I.M.; Riihonen, T. (2020). «Coefficients for Global Minimax Approximations and Bounds for the Gaussian Q-Function by Sums of Exponentials [Data set]». Zenodo. doi:10.5281/zenodo.4112978.
- ^ Karagiannidis, G. K.; Lioumpas, A. S. (2007). «An improved approximation for the Gaussian Q-function» (PDF). IEEE Communications Letters. 11 (8): 644–646. doi:10.1109/LCOMM.2007.070470. S2CID 4043576.
- ^ Tanash, I.M.; Riihonen, T. (2021). «Improved coefficients for the Karagiannidis–Lioumpas approximations and bounds to the Gaussian Q-function». IEEE Communications Letters. 25 (5): 1468–1471. arXiv:2101.07631. doi:10.1109/LCOMM.2021.3052257. S2CID 231639206.
- ^ Chang, Seok-Ho; Cosman, Pamela C.; Milstein, Laurence B. (November 2011). «Chernoff-Type Bounds for the Gaussian Error Function». IEEE Transactions on Communications. 59 (11): 2939–2944. doi:10.1109/TCOMM.2011.072011.100049. S2CID 13636638.
- ^ Winitzki, Sergei (2003). «Uniform approximations for transcendental functions». Computational Science and Its Applications – ICCSA 2003. Lecture Notes in Computer Science. Vol. 2667. Springer, Berlin. pp. 780–789. doi:10.1007/3-540-44839-X_82. ISBN 978-3-540-40155-1.
- ^ Zeng, Caibin; Chen, Yang Cuan (2015). «Global Padé approximations of the generalized Mittag-Leffler function and its inverse». Fractional Calculus and Applied Analysis. 18 (6): 1492–1506. arXiv:1310.5592. doi:10.1515/fca-2015-0086. S2CID 118148950.
Indeed, Winitzki [32] provided the so-called global Padé approximation
- ^ Winitzki, Sergei (6 February 2008). «A handy approximation for the error function and its inverse» (Document).
- ^ Numerical Recipes in Fortran 77: The Art of Scientific Computing (ISBN 0-521-43064-X), 1992, page 214, Cambridge University Press.
- ^ Dia, Yaya D. (2023). Approximate Incomplete Integrals, Application to Complementary Error Function. Available at SSRN: https://ssrn.com/abstract=4487559 or http://dx.doi.org/10.2139/ssrn.4487559, 2023
- ^ a b c Cody, W. J. (March 1993), «Algorithm 715: SPECFUN—A portable FORTRAN package of special function routines and test drivers» (PDF), ACM Trans. Math. Softw., 19 (1): 22–32, CiteSeerX 10.1.1.643.4394, doi:10.1145/151271.151273, S2CID 5621105
- ^ Zaghloul, M. R. (1 March 2007), «On the calculation of the Voigt line profile: a single proper integral with a damped sine integrand», Monthly Notices of the Royal Astronomical Society, 375 (3): 1043–1048, Bibcode:2007MNRAS.375.1043Z, doi:10.1111/j.1365-2966.2006.11377.x
- ^ John W. Craig, A new, simple and exact result for calculating the probability of error for two-dimensional signal constellations Archived 3 April 2012 at the Wayback Machine, Proceedings of the 1991 IEEE Military Communication Conference, vol. 2, pp. 571–575.
- ^ Behnad, Aydin (2020). «A Novel Extension to Craig’s Q-Function Formula and Its Application in Dual-Branch EGC Performance Analysis». IEEE Transactions on Communications. 68 (7): 4117–4125. doi:10.1109/TCOMM.2020.2986209. S2CID 216500014.
- ^ Carslaw, H. S.; Jaeger, J. C. (1959), Conduction of Heat in Solids (2nd ed.), Oxford University Press, ISBN 978-0-19-853368-9, p 484
- ^ «math.h — mathematical declarations». opengroup.org. 2018. Retrieved 21 April 2023.
- ^ «Special Functions – GSL 2.7 documentation».
Further reading[edit]
- Abramowitz, Milton; Stegun, Irene Ann, eds. (1983) [June 1964]. «Chapter 7». Handbook of Mathematical Functions with Formulas, Graphs, and Mathematical Tables. Applied Mathematics Series. Vol. 55 (Ninth reprint with additional corrections of tenth original printing with corrections (December 1972); first ed.). Washington D.C.; New York: United States Department of Commerce, National Bureau of Standards; Dover Publications. p. 297. ISBN 978-0-486-61272-0. LCCN 64-60036. MR 0167642. LCCN 65-12253.
- Press, William H.; Teukolsky, Saul A.; Vetterling, William T.; Flannery, Brian P. (2007), «Section 6.2. Incomplete Gamma Function and Error Function», Numerical Recipes: The Art of Scientific Computing (3rd ed.), New York: Cambridge University Press, ISBN 978-0-521-88068-8
- Temme, Nico M. (2010), «Error Functions, Dawson’s and Fresnel Integrals», in Olver, Frank W. J.; Lozier, Daniel M.; Boisvert, Ronald F.; Clark, Charles W. (eds.), NIST Handbook of Mathematical Functions, Cambridge University Press, ISBN 978-0-521-19225-5, MR 2723248.
External links[edit]
- A Table of Integrals of the Error Functions
Use this ERF calculator to easily calculate the Gauss error function erf(x) for any real-valued x and the inverse error function erf-1(y), y ∈ [-1, 1]. It can also output their complementary functions erfc(x) and erfc-1(y). High-precision calculation up to 25 significant digits. A table of x values and corresponding values of erf and erfc is included as reference.
Quick navigation:
- Using the error function calculator
- What is an error function?
- Error function formula
- Complementary error function
- Inverse error function
- Error function table
- Example application
Using the error function calculator
The erf calculator can be used to compute the error function of any number on the real line. The output also contains the complementary error function for the same number as well as a function plot showing where erf(x) lies relative to other possible function values. erf(x) returns a result between zero and one for any real value of x.
In inverse error function mode the output contains the inverse of erf and its complement. The input y needs to be a real number between minus one and one (y ∈ [-1, 1]).
What is an error function?
In mathematics and statistics, the error function a.k.a. the Gauss error function or just erf, is a complex function of a complex variable defined as [1,2]:
Aside from applied mathematics where it is used to solve differential equations and in physics in solutions of the heat equation in the case where boundary conditions are given by the Heaviside step function, it also sees use in statistics where the inverse error function is used in the calculation of critical values, p-values, confidence intervals which are all related to statistical hypothesis testing and estimation.
The function plot illustrates its sigmoid shape:
Since e-t2 is an even function and erf(-x) = -erf(x), the error function is an odd function.
Error function formula
To calculate erf(x) one performs an integration from minus infinity to x of the equation e-t2. The formula can therefore be expressed by the following integral equation:
The equation has no closed-form solution and various approximations are in use. In this calculator we use a polynomial approximation with a maximal error of 1.2 × 107 for any real argument as per reference [3].
Complementary error function
The equation for the complementary error function is given by:
Its solution is a simple subtraction from one. It sees application in physics problems.
Inverse error function
The inverse error function, denoted erf-1(y) takes as input the result of y = erf(x), and produces the corresponding x value. While a true inverse function would be multivalued and thus would not have a unique solution, for values of y between −1 and 1 there is a unique real number solution to the equation:
The solution can easily be found by various root-finding methods.
Error function table
An erf table contains tabulated values of real numbers and their corresponding error function values.
Table of commonly used numbers:
| x | erf(x) | erfc(x) |
|---|---|---|
| 0.000 | 0.000000 | 1.000000 |
| 0.01 | 0.011283 | 0.988717 |
| 0.02 | 0.022565 | 0.977435 |
| 0.05 | 0.056372 | 0.943628 |
| 0.10 | 0.112463 | 0.887537 |
| 0.20 | 0.222703 | 0.777297 |
| 0.30 | 0.328627 | 0.671373 |
| 0.40 | 0.428392 | 0.571608 |
| 0.50 | 0.520500 | 0.479500 |
| 0.60 | 0.603856 | 0.396144 |
| 0.70 | 0.677801 | 0.322199 |
| 0.80 | 0.742101 | 0.257899 |
| 0.90 | 0.796908 | 0.203092 |
| 1.00 | 0.842701 | 0.157299 |
| 1.10 | 0.880205 | 0.119795 |
| 1.20 | 0.910314 | 0.089686 |
| 1.30 | 0.934008 | 0.065992 |
| 1.40 | 0.952285 | 0.047715 |
| 1.50 | 0.966105 | 0.033895 |
| 1.60 | 0.976348 | 0.023652 |
| 1.70 | 0.983790 | 0.016210 |
| 1.80 | 0.989091 | 0.010909 |
| 1.90 | 0.992790 | 0.007210 |
| 2.00 | 0.995322 | 0.004678 |
| 2.25 | 0.998537 | 0.001463 |
| 2.50 | 0.999593 | 0.000407 |
| 2.75 | 0.999899 | 0.000101 |
| 3.00 | 0.999978 | 0.000022 |
| 3.50 | 0.999999 | 0.000001 |
| 4.00 | 1.000000 | 0.000000 |
Example application
The inverse error function can be used to compute the quantile function (inverse distribution function) of a normal distribution. For example, if we want to find the cut-off for the top decile of a normal distribution with mean μ = 0 and standard deviation σ = 3, we would use the equation:
F-1(0.9) = μ + σ · √2 · erf-1(2 · p — 1)
Knowing that the top decile represents the top 10 percent, we need to find the cut-off at 90%, or 0.9. Replacing the known values we get:
F-1(0.9) = 0 + 3 · √2 · erf-1(2 · 0.9 — 1) = 3 · √2 · erf-1(0.8)
Using a square root calculator we find √2 equals 1.4142 and using this calculator we find erf-1(0.8) = 0.9062. Replacing once again we find the solution:
F-1(0.9) = 3 · 1.4142 · 0.9062 = 3.8446 which is accurate to four significant digits, same as the accuracy of all numbers used in obtaining the result.
References
1 Glaisher, J.W.L. (1871) «On a class of definite integrals» London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science 4 42(277): 294–302. DOI 10.1080/14786447108640568
2 Glaisher, J.W.L. (1871) «On a class of definite integrals. Part II.» London, Edinburgh, and Dublin Philosophical Magazine and Journal of Science 4 42(279): 421–426. DOI 10.1080/14786447108640600
3 «Numerical Recipes in Fortran 77: The Art of Scientific Computing» (1992), page 214, Cambridge University Press ISBN 0-521-43064-X
Created by Anna Szczepanek, PhD
Reviewed by
Dominik Czernia, PhD and Jack Bowater
Last updated:
Jun 05, 2023
Welcome to the error function calculator! It helps you compute the values of the four function from the erf family:
- Error function itself;
- Complementary error function;
- Inverse error function; and
- Inverse complementary error function.
If you are not sure what the Gaussian error function actually is, don’t worry! If you scroll down, you will find all the necessary definitions and plots, as well as a short explanation of why the error function matters. As a bonus, we will show you how to (approximately) calculate erf by hand! Finally, at the very bottom of the page, you can find the error function table.
What is the error function?
The error function (often abbreviated to erf, also known as the Gaussian error function) is a special function that we encounter in applied mathematics and mathematical physics, e.g., in solutions to the heat equation.
For a real number x, the erf function is defined as
erf(x)=2π∫0xexp(−t2)dt\small
\textrm{erf}(x) = \frac 2{\sqrt{\pi}}\int_0^x\operatorname{exp}(-t^2)\textrm{d}t
In the plot below, we see that erf is an odd sigmoid function.
In statistics and probability theory, the error function has the following interpretation: for a random variable Z that follows the normal distribution with mean 0 and variance 0.5, the probability that Z falls in the interval [−x, x] is equal to erf(x), where we assume that x is non-negative. As a consequence, the error function is involved in many different calculations, in particular those you can encounter in the following Omni tools:
- p-value calculator;
- Confidence intervals calculator; and
- Critical values calculator.
Complementary error function
The complementary error function, most often denoted by erfc, is defined as one minus the error function. That is, we have
erfc(x)=1−erf(x)\textrm{erfc}(x) = 1 — \textrm{erf}(x)
which we can also write as
erfc(x)=2π∫x∞exp(−t2)dt\small
\textrm{erfc}(x) = \frac 2{\sqrt{\pi}}\int_x^\infty\operatorname{exp}(-t^2)\textrm{d}t
In statistics, the complementary error function erfc(x), where we assume that x is non-negative, describes the probability that a random variable Z following the Gaussian normal distribution with mean 0 and variance 0.5 falls outside the interval [−x, x].
Inverse error function
As we can see from the plot of the error function, if −1 < x < 1, then there exists a unique real number denoted erf-1(x), such that:
erf(erf−1(x))=x.\textrm{erf}(\textrm{erf}^{-1}(x)) =x.
In this way we obtain the inverse error function. Below you can see its plot:
Finally, there is the inverse complementary error function erfc-1, which we define as
erfc−1(x)=erf−1(1−x).\textrm{erfc}^{-1}(x) = \textrm{erf}^{-1}(1-x).
How to calculate erf using this error function calculator?
As the error function is a non-elementary function (as are the other three functions we defined above with the help of erf), it’s not easy to find their values for a given argument x. Fortunately, Omni’s erf calculator is here to help!
-
In the
modefield, choose which of the four functions from the erf family you want to calculate. -
Enter the value of the argument at which you want the function evaluated.
-
Our error function calculator returns the answer immediately. Enjoy!
How to calculate erf by hand?
What if one day you need to determine the Gaussian error function without a dedicated calculator at hand? Your situation isn’t hopeless. There are several good ways of approximating erf. Let us mention two of them.
- The error function has the following Taylor (Maclaurin) series:
erf(x)=2π∑n=0∞(−1)nx2n+1(2n+1)n!=2π(x−x33+x510−x742+…)\footnotesize
\begin{align*}
\textrm{erf}(x) & = \frac 2{\sqrt{\pi}}\sum_{n=0}^\infty \frac{(-1)^n x^{2n+1}}{(2n+1)n!}\\
& = \frac 2{\sqrt{\pi}}\left(\!x \!-\! \frac{x^3}{3} \!+\! \frac{x^5}{10} \!-\! \frac{x^7}{42} \!+\! \ldots \! \right)
\end{align*}
It holds for all real arguments x. In practical applications, you need to compute a partial sum of this series, i.e., the sum of several initial terms. The more, the better the approximation.
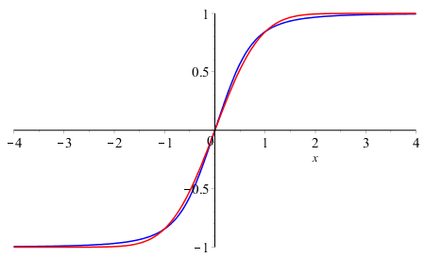
- It turns out that a suitably transformed cyclometric (inverse trigonometric) function,
arctan, can serve as a quite good approximation of the error function:
erf(x)≈2πarctan(2x(1+x4))\small
\textrm{erf}(x) \approx \frac 2{{\pi}}\operatorname{arctan}(2x(1+x^4))
The plot below shows these two functions so that you can see how good the approximation is.
Error function table
Since erf is a special function and cannot be easily calculated without a dedicated calculator, there’s been a long tradition of tabulating its values. In case you ever need such a table, we give it below. It covers the arguments between 0 and 3. For negative arguments you need to utilize the fact that erf is an odd function, i.e., that erf(-x) = -erf(x).
|
x |
erf(x) |
erfc(x) |
|---|---|---|
|
0 |
0 |
1 |
|
0.01 |
0.011283416 |
0.988716584 |
|
0.02 |
0.022564575 |
0.977435425 |
|
0.03 |
0.033841222 |
0.966158778 |
|
0.04 |
0.045111106 |
0.954888894 |
|
0.05 |
0.056371978 |
0.943628022 |
|
0.06 |
0.067621594 |
0.932378406 |
|
0.07 |
0.07885772 |
0.92114228 |
|
0.08 |
0.090078126 |
0.909921874 |
|
0.09 |
0.101280594 |
0.898719406 |
|
0.1 |
0.112462916 |
0.887537084 |
|
0.2 |
0.222702589 |
0.777297411 |
|
0.3 |
0.328626759 |
0.671373241 |
|
0.4 |
0.428392355 |
0.571607645 |
|
0.5 |
0.520499878 |
0.479500122 |
|
0.6 |
0.603856091 |
0.396143909 |
|
0.7 |
0.677801194 |
0.322198806 |
|
0.8 |
0.742100965 |
0.257899035 |
|
0.9 |
0.796908212 |
0.203091788 |
|
1 |
0.842700793 |
0.157299207 |
|
1.1 |
0.88020507 |
0.11979493 |
|
1.2 |
0.910313978 |
0.089686022 |
|
1.3 |
0.934007945 |
0.065992055 |
|
1.4 |
0.95228512 |
0.04771488 |
|
1.5 |
0.966105146 |
0.033894854 |
|
1.6 |
0.976348383 |
0.023651617 |
|
1.7 |
0.983790459 |
0.016209541 |
|
1.8 |
0.989090502 |
0.010909498 |
|
1.9 |
0.992790429 |
0.007209571 |
|
2 |
0.995322265 |
0.004677735 |
|
2.5 |
0.999593048 |
0.000406952 |
|
3 |
0.99997791 |
0.00002209 |
|
3.5 |
0.999999257 |
0.000000743 |
Absolute value equationAbsolute value inequalitiesAdding and subtracting polynomials… 37 more


}{{\sqrt%20%20\pi%20}}})






![{\displaystyle {\begin{aligned}\Pr[X\leq L]&={\frac {1}{2}}+{\frac {1}{2}}\operatorname {erf} {\frac {L-\mu }{{\sqrt {2}}\sigma }}\\&\approx A\exp \left(-B\left({\frac {L-\mu }{\sigma }}\right)^{2}\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/f3cb760eaf336393db9fd0bb12c4465655a27de8)
![{\displaystyle \Pr[X\leq L]\leq A\exp(-B\ln {k})={\frac {A}{k^{B}}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2baadea015e20a45d1034fd88eed861e7fcce178)
![{\displaystyle {\begin{aligned}\Pr[L_{a}\leq X\leq L_{b}]&=\int _{L_{a}}^{L_{b}}{\frac {1}{{\sqrt {2\pi }}\sigma }}\exp \left(-{\frac {(x-\mu )^{2}}{2\sigma ^{2}}}\right)\,\mathrm {d} x\\&={\frac {1}{2}}\left(\operatorname {erf} {\frac {L_{b}-\mu }{{\sqrt {2}}\sigma }}-\operatorname {erf} {\frac {L_{a}-\mu }{{\sqrt {2}}\sigma }}\right).\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/cd2214f0db2c1d36075815825b616501175c6283)


![{\displaystyle {\begin{aligned}\operatorname {erf} z&={\frac {2}{\sqrt {\pi }}}\sum _{n=0}^{\infty }{\frac {(-1)^{n}z^{2n+1}}{n!(2n+1)}}\\[6pt]&={\frac {2}{\sqrt {\pi }}}\left(z-{\frac {z^{3}}{3}}+{\frac {z^{5}}{10}}-{\frac {z^{7}}{42}}+{\frac {z^{9}}{216}}-\cdots \right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c80541f305af070bb0510625c584fe1559a0cd2c)
![{\displaystyle {\begin{aligned}\operatorname {erf} z&={\frac {2}{\sqrt {\pi }}}\sum _{n=0}^{\infty }\left(z\prod _{k=1}^{n}{\frac {-(2k-1)z^{2}}{k(2k+1)}}\right)\\[6pt]&={\frac {2}{\sqrt {\pi }}}\sum _{n=0}^{\infty }{\frac {z}{2n+1}}\prod _{k=1}^{n}{\frac {-z^{2}}{k}}\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/dca22e8e7dee0297e87a455249c282c6b92fedcb)
![{\displaystyle {\begin{aligned}\operatorname {erfi} z&={\frac {2}{\sqrt {\pi }}}\sum _{n=0}^{\infty }{\frac {z^{2n+1}}{n!(2n+1)}}\\[6pt]&={\frac {2}{\sqrt {\pi }}}\left(z+{\frac {z^{3}}{3}}+{\frac {z^{5}}{10}}+{\frac {z^{7}}{42}}+{\frac {z^{9}}{216}}+\cdots \right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/2ff91095cd6825137cc951ec0a786db0b7f68fac)





![{\displaystyle {\begin{aligned}\operatorname {erf} x&={\frac {2}{\sqrt {\pi }}}\operatorname {sgn} x\cdot {\sqrt {1-e^{-x^{2}}}}\left(1-{\frac {1}{12}}\left(1-e^{-x^{2}}\right)-{\frac {7}{480}}\left(1-e^{-x^{2}}\right)^{2}-{\frac {5}{896}}\left(1-e^{-x^{2}}\right)^{3}-{\frac {787}{276480}}\left(1-e^{-x^{2}}\right)^{4}-\cdots \right)\\[10pt]&={\frac {2}{\sqrt {\pi }}}\operatorname {sgn} x\cdot {\sqrt {1-e^{-x^{2}}}}\left({\frac {\sqrt {\pi }}{2}}+\sum _{k=1}^{\infty }c_{k}e^{-kx^{2}}\right).\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/164e7f029977edb47c83845b04abfe5b2d28b837)







![{\displaystyle {\begin{aligned}\operatorname {erfc} x&={\frac {e^{-x^{2}}}{x{\sqrt {\pi }}}}\left(1+\sum _{n=1}^{\infty }(-1)^{n}{\frac {1\cdot 3\cdot 5\cdots (2n-1)}{\left(2x^{2}\right)^{n}}}\right)\\[6pt]&={\frac {e^{-x^{2}}}{x{\sqrt {\pi }}}}\sum _{n=0}^{\infty }(-1)^{n}{\frac {(2n-1)!!}{\left(2x^{2}\right)^{n}}},\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/35a11e2e5b22ca898c74f2e913d276c9ac11124a)
































![{\displaystyle {\begin{aligned}\operatorname {erfc} x&=1-\operatorname {erf} x\\[5pt]&={\frac {2}{\sqrt {\pi }}}\int _{x}^{\infty }e^{-t^{2}}\,\mathrm {d} t\\[5pt]&=e^{-x^{2}}\operatorname {erfcx} x,\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/4acd0062271e2a19c209a02c8cc33d44a28af7cc)



![{\displaystyle {\begin{aligned}\operatorname {erfi} x&=-i\operatorname {erf} ix\\[5pt]&={\frac {2}{\sqrt {\pi }}}\int _{0}^{x}e^{t^{2}}\,\mathrm {d} t\\[5pt]&={\frac {2}{\sqrt {\pi }}}e^{x^{2}}D(x),\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/bfd2dd94cd6d0325224d412f6b5e5ed63ca81d4a)


![{\displaystyle {\begin{aligned}\Phi (x)&={\frac {1}{\sqrt {2\pi }}}\int _{-\infty }^{x}e^{\tfrac {-t^{2}}{2}}\,\mathrm {d} t\\[6pt]&={\frac {1}{2}}\left(1+\operatorname {erf} {\frac {x}{\sqrt {2}}}\right)\\[6pt]&={\frac {1}{2}}\operatorname {erfc} \left(-{\frac {x}{\sqrt {2}}}\right)\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/89a9e9eaaddcd7a91ade15a41b8d1e272d437559)
![{\displaystyle {\begin{aligned}\operatorname {erf} (x)&=2\Phi \left(x{\sqrt {2}}\right)-1\\[6pt]\operatorname {erfc} (x)&=2\Phi \left(-x{\sqrt {2}}\right)\\&=2\left(1-\Phi \left(x{\sqrt {2}}\right)\right).\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/86c84a4d2d79631fe9996e30f1d6c0da3089bfe2)








![{\displaystyle {\begin{aligned}\operatorname {i} ^{n}\!\operatorname {erfc} z&=\int _{z}^{\infty }\operatorname {i} ^{n-1}\!\operatorname {erfc} \zeta \,\mathrm {d} \zeta \\[6pt]\operatorname {i} ^{0}\!\operatorname {erfc} z&=\operatorname {erfc} z\\\operatorname {i} ^{1}\!\operatorname {erfc} z&=\operatorname {ierfc} z={\frac {1}{\sqrt {\pi }}}e^{-z^{2}}-z\operatorname {erfc} z\\\operatorname {i} ^{2}\!\operatorname {erfc} z&={\tfrac {1}{4}}\left(\operatorname {erfc} z-2z\operatorname {ierfc} z\right)\\\end{aligned}}}](https://wikimedia.org/api/rest_v1/media/math/render/svg/c3e7b953efaa4d730a5479bd61a2c378c8f761dc)